Write-up 7: Tangent Circles and a Proof Accessible to High School Students

This write-up is based on the following prompt:

Given two circles and a point on one of the circles, construct a circle tangent to the two circles with one point of tangency being the designated point.

Though there are three different cases for such circles - one circle inside the other, two intersecting circles, and two disjoint circles outside the interior of each other - we will demonstrate the construction of the inner tangent circle in the first case. The construction is as follows:

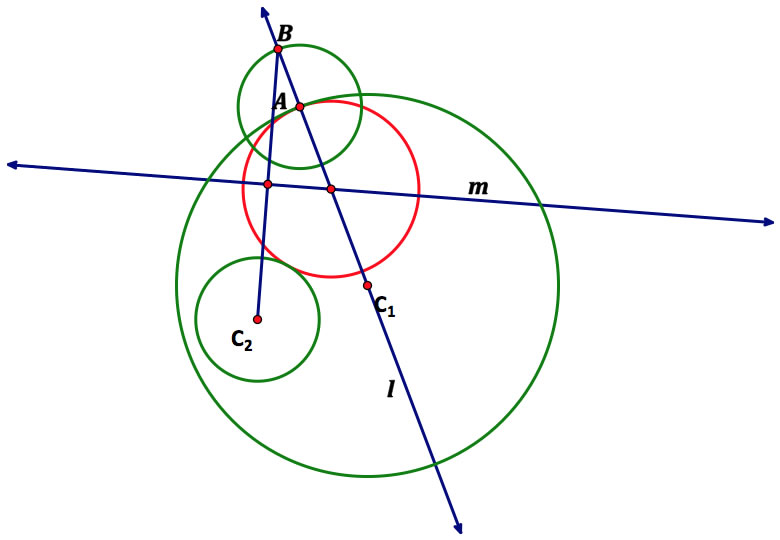
The following is a GSP tool for tangent circles. (Titled "Blue and Red Tangent Circles*)

One interesting consideration is the loci of the center of both tangent circles in the first case.

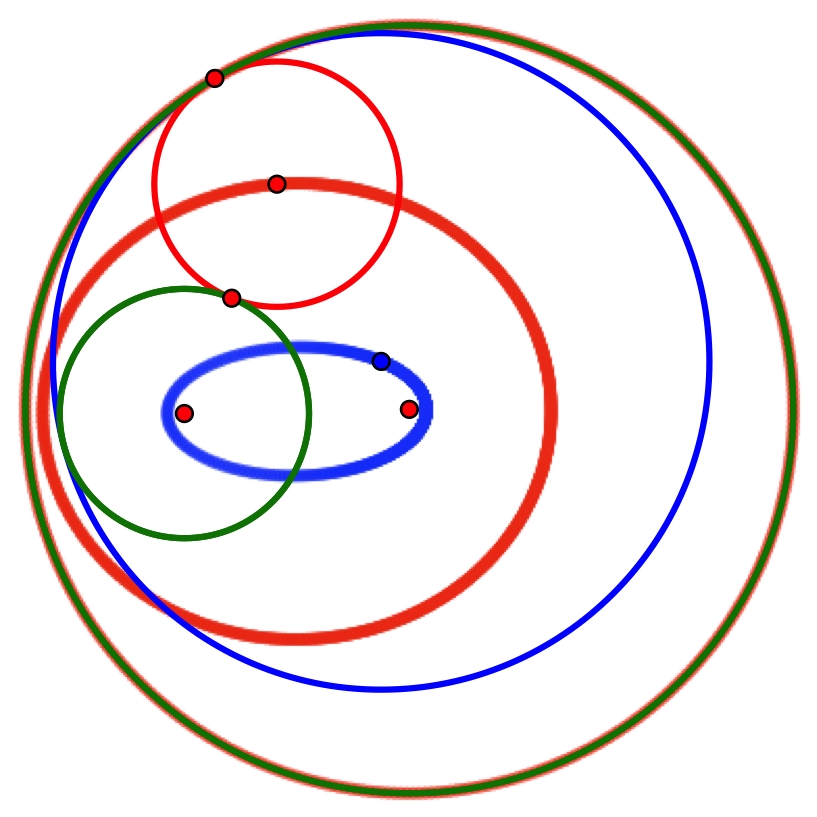
It appears both loci are ellipses. Indeed, we can justify as follows:

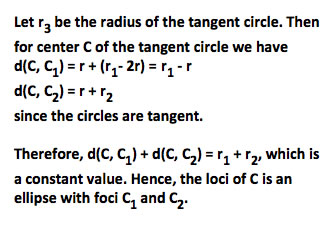
The proof is similar for the outer tangent circle.

In the case of two intersecting circles, we have that the path of the center of the inner tangent circle remains an ellipse and the center of the outer tangent circle follows the path of a hyperbola. We will not justify here.

In the case of two disjoint circles that are not located in the interior of the other, then the loci of both centers are hyperbolas. Again, we will not justify here.

 

The second part of this write-up focuses on the locus of a different point: the midpoint of B and the center of the smaller tangent circle. By tracing this point in GSP, the locus of midpoint G appears to be a circle...

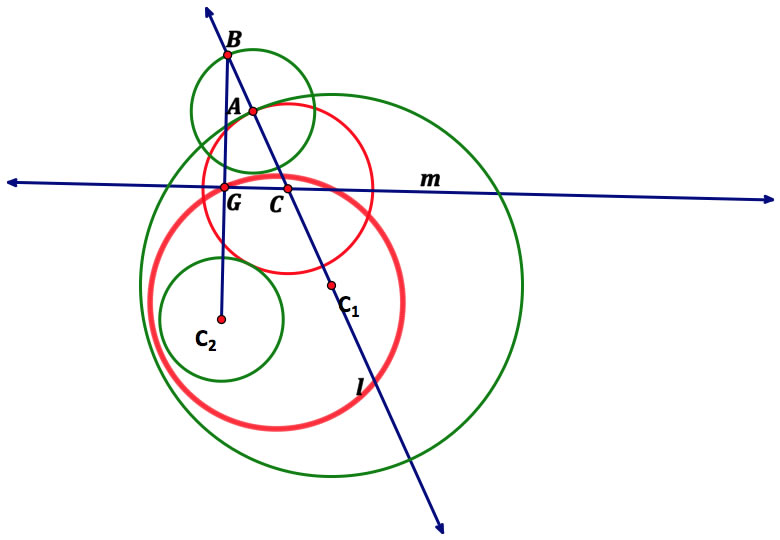
The justification of this proof requires a deceptively simple geometry theorem that is currently in the Common Core Georgia Performance Standards: the Triangle Midsegment Theorem. Consider the following isolated part of the above diagram:

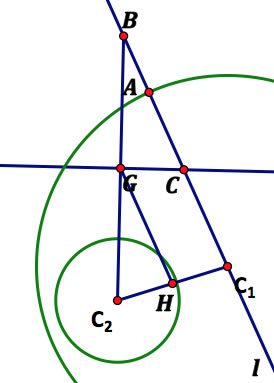
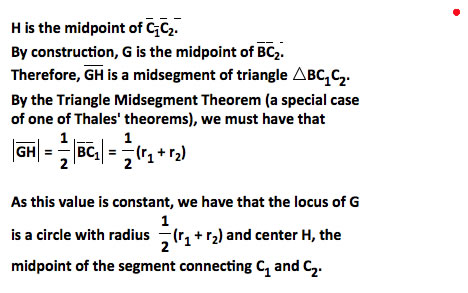
It was striking to me, that two days prior to my investigating the locus of this point, I had taught the midsegment theorem to tenth grade students - teaching them that the midsegment is half the length of the base or "third side" and parallel to this side. Though this construction requires some abstract thinking and comfortability with variables that the average tenth grade student might not have, it more than makes up for its difficulty in the enjoyment that discovering its result provides. Perhaps such an investigation could be incorporated in a unit on constructions after students have already mastered the midsegment theorem. Regardless, seeing current high school mathematics be not only involved but integrally useful in collegiate explorations makes teaching it all the more relevant and fulfilling.